Seabed sand waves
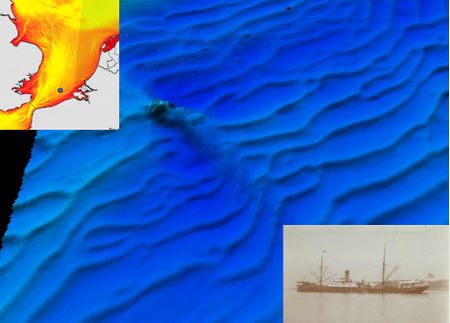
Seabed sand waves are more or less rectilinear sand ridges arising from the interaction of tidal flow with the seabed. They form a regular undulating pattern with wavelengths (=distance between successive crests) of one hundred to one thousand meters, but most often between 200 and 700 m. Seabed sand waves can become quite high, up to half the water depth. They can therefore form an obstacle to shipping. Double-crested sand waves of much larger wavelengths (more than twice the single-crested sand waves) have also been observed[1].
Seabed sand waves are a ubiquitous feature of sandy continental shelves with significant tidal currents. Seabed sand waves should not be confused with shoreline sand waves (see Rhythmic shoreline features), which are a completely different phenomenon. Since seabed sand waves are akin to river dunes, a more appropriate name would be tidal dunes.
Contents
[hide]Occurrence of sand waves
Sand waves are formed spontaneously when sediment particles are dragged back and forth over the seabed by tidal currents. The formation process is similar to the formation process of bottom ripples by wave action. Sand waves generate and are generated by tide-averaged circulation cells. The development of both is a mutually reinforcing process, as explained in the article Principles of spontaneous bedform generation.
Sufficient strength of tidal currents to pick up and transport sediment particles is a first requirement for the occurrence of sand waves. A coarse sediment bed requires a larger current strength than a sediment bed consisting of fine sand. A second requirement concerns the phase lag of the transported sediment load with respect to the phase of the tidal current. This phase lag must be small to ensure that maximum sediment transport occurs upstream of the sand wave crest. This is the case if the tide is not strongly asymmetric and if bedload transport dominates over suspended load transport. Bedload is dominant when the bottom friction velocity of the tidal current [math]u*[/math] (representative of the magnitude of turbulent velocity fluctuations near the seabed) does not greatly exceed the average settling velocity [math]w_s[/math] of sediment particles. For example, the maximum tidal velocity in 40 m deep water should not exceed 0.5-0.6 m/s in the case of fine sand (average grain size 0.2 mm), see Sand transport. Seabed sand waves occur when sediment grainsize and tidal current strength meet these conditions. No sand waves have been observed in situations where suspended sediment transport dominates over bedload transport. This is consistent with the results of numerical model simulations, which show that suspended load transport and tidal asymmetry each have a significant dampening effect on the equilibrium wave height[2].
Description of sand waves
The wavelength of sand waves is much smaller than the distance over which sediment particles are dragged over the seabed during a tidal period. This contrasts with the wavelength of seabed ripples, which is of the same order of magnitude as the horizontal wave orbital excursion. The reason is that the secondary circulations which drag sediment particles from the trough zone to the crest zone are weak when trough and crest are far apart, while the distance over which sediment particles must be dragged is large. It has been suggested that large sand waves grow by coalescence of smaller ones, as has also been observed for wave ripples[3].
The wavelength of river dunes, which are generated by a similar process as sand waves (tidal dunes), is positively correlated with the water depth. The wavelength of sand waves also increases with increasing water depth, but probably only up to a certain limit. The secondary circulations responsible for the growth of river dunes and sand waves are a boundary layer phenomenon. The duration of the ebb and flood periods limit the vertical diffusion of eddies generated by bottom friction over the water column. In deep water, the logarithmic tidal boundary layer therefore does reach the water surface[4]. It may thus be expected that the generation of sand waves in deep water does not depend on the water depth[5].
The height of sand waves is positively correlated with the wavelength. The relationship [math]H_{mean} \approx 0.07 \, L^{0.8}[/math] was found from a large number of observations of different sand wave fields, where [math]H_{mean}[/math] is the mean crest height and [math]L[/math] the wavelength[5]. However, if the thickness of the erodible sediment layer is small, the sand wave will not fully develop[6]. This is because sediment for crest growth is supplied by scouring of the sand wave trough.
The sand wave height is also influenced by waves. Strong waves decrease the growth rate of sand waves by enhancing downslope gravitational transport[7]. Moreover, the shape of sand waves is affected by the wind-wave climate. Flat-topped three-dimensional sand waves are observed in shallow waters, where sea waves give a significant contribution to the local hydrodynamics even close to the bottom, whereas two-dimensional sand waves are observed farther offshore where sea waves do not induce any significant water motion close to the sea bed[8]. Storms tend to reduce growth rates, in a way that favors sand waves with longer wavelengths than those generated during fair weather conditions[9].
Sand wave migration
Sand waves are stationary in the absence of residual currents and when tides are symmetric. Since this ideal situation is rarely met, sand waves generally migrate. For the continental shelf off the Netherlands, for example, typical migration rates are between 0 and 3 m/year. The highest migration rates are observed near the coast and on the top of sandbanks[10]. Sand waves have an asymmetric shape when the tidal wave is asymmetric or when a residual current is present. In these cases, sand waves migrate at a typical rate of some tens of meters per year. Stirring due to wind waves also enhances sand wave migration[8]. Wind-driven flow in combination with waves may result in significant migration rates during storms, even reversing the fair weather migration[11].
Risks of sand waves to marine activities
By reducing the local water depth, sand waves form an obstacle to large ships, so intensive dredging may be required. Sand wave migration can expose objects buried in the seabed, increasing the risk of hazardous substances from sunken ships, mines or containers being released into the seawater. Pipelines laid on or in the seabed may end up in a free span position due to migration of sand waves. Migrating sand waves can also undermine the foundations of offshore structures.[8]
Related articles
References
- Jump up ↑ Zhou, J., Wu, Z., Zhao, D., Guan, W., Zhu, C. and Flemming, B. 2020. Giant sand waves on the Taiwan Banks, southern Taiwan Strait: Distribution, morphometric relationships, and hydrologic influence factors in a tide-dominated environment. Marine Geology 427, 106238
- Jump up ↑ van Gerwen, W., Borsje, B.W., Damveld, J.H. and Hulscher S.J.M.H. 2018. Modelling the effect of suspended load transport and tidal asymmetry on the equilibrium tidal sand wave height. Coastal Engineering 136: 56–64
- Jump up ↑ Flemming, B.W. 2000. On the dimensional adjustment of subaqueous dunes in response to changing flow conditions: a conceptual process model. In: Trentesaux, A., Garlan, T. (Eds.), Marine Sandwave Dynamics. Proceedings International Workshop, University of Lille, pp. 61–67
- Jump up ↑ Lueck, R.G. and Lu, Y. 1997. The logarithmic layer in a tidal channel. Continental Shelf Research 17: 1785-1801
- ↑ Jump up to: 5.0 5.1 Flemming, B.W. 2000. The role of grain size, water depth and flow velocity as scaling factors controlling the size of subaqueous dunes. In: Trentesaux, A., Garlan, T. (Eds.), Marine Sandwave Dynamics. Proceedings International Workshop, University of Lille, pp. 55–60
- Jump up ↑ Nnafie, A., Krabbendam, J.M. and de Swart, H.E. 2023. Consequences of limited sediment supply for long-term evolution of offshore tidal sand waves, a 3D model perspective. Marine Geology 464, 107148
- Jump up ↑ Campmans, G.H.P., Roos, P.C., de Vriend, H.J. and Hulscher S.J.M.H. 2017. Modeling the influence of storms on sand wave formation: A linear stability Approach. Continental Shelf Research 137: 103–116
- ↑ Jump up to: 8.0 8.1 8.2 Vittori, G. and Blondeaux, P. 2022. Predicting offshore tidal bedforms using stability methods. Earth-Sci. Rev. 235, 104234
- Jump up ↑ Campmans, G.H.P., Roos, P.C., de Vriend, H.J. and Hulscher S.J.M.H. 2017. Modeling the influence of storms on sand wave formation: A linear stability Approach. Continental Shelf Research 137: 103–116
- Jump up ↑ Van der Meijden, R., Damveld, J.H., Ecclestone, D.W., Van der Werf, J.J. and Roos, P.C. 2023. Shelf-wide analyses of sand wave migration using GIS: A case study on the Netherlands Continental Shelf. Geomorphology 424, 108559
- Jump up ↑ Campmans, G.H.P., Roos, P.C., de Vriend, H.J. and Hulscher S.J.M.H. 2017. Modeling the influence of storms on sand wave formation: A linear stability Approach. Continental Shelf Research 137: 103–116
Please note that others may also have edited the contents of this article.
|