Wave run-up
Definition of Wave run-up:
Wave run-up is the maximum onshore elevation reached by waves, relative to the shoreline position in the absence of waves.
This is the common definition for Wave run-up, other definitions can be discussed in the article
|
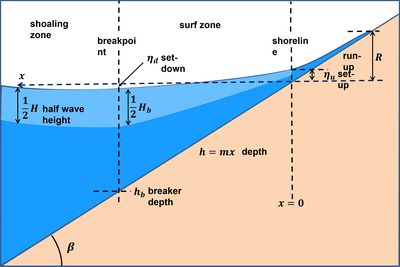
Wave run-up is the sum of wave set-up and swash uprush and must be added to the water level reached as a result of tides and wind set-up (Fig. 1). Wave run-up on a beach is generally due to so-called swash bores: the uprush of waves after final collapse on the beach. Wave run-up is an important parameter for assessing the safety of sea dikes or coastal settlements.
Waves refer to waves generated by wind (locally or on the ocean) or waves generated by incidental disturbances of the sea surface such as tsunamis, seiches or ship waves. Wave run-up is often indicated with the symbol [math] R [/math].
Contents
[hide]Empirical formulas sandy beaches
For waves collapsing on the beach, a first order-of-magnitude estimate is given by the empirical formula of Hunt (1959) [1][2][3],
[math]R \sim \eta_u + H \xi , \qquad (1)[/math]
where [math]\eta_u \sim 0.2 H[/math] is the wave set-up, [math]H[/math] is the offshore significant wave height and [math]\xi[/math] is the surf similarity parameter,
[math]\xi = \Large\frac{\beta}{\sqrt{H/L}}\normalsize = T \, \beta \, \Large\sqrt{\frac{g}{2\pi H}}\normalsize , \qquad (2)[/math]
where [math]\tan \beta \approx \beta[/math] is the beach slope and [math]T[/math] is the peak wave period. The offshore wavelength [math]L[/math] is given by [math]L = g T^2/(2 \pi)[/math], assuming that [math]L / (2 \pi) [/math] is smaller than the local depth [math]h[/math]. The horizontal wave incursion is approximately given by [math] R / \beta[/math].
The maximum run-up depends on the mean water level at the shoreline. This water level is increased by wave-induced setup [math]\eta_0[/math], see Wave set-up. The following empirical relationship has been derived for the sum of maximum wave setup and swash runup[4]:
[math] R \approx 0.73 \; \beta \; \sqrt{HL} . \qquad (3)[/math]
Many empirical formulas have been proposed for the run-up. A popular formula for the run-up [math] R_2[/math] exceeded by only 2 % of the waves for given [math]H, L[/math] has been developed by Stockdon et al. (2006[4]), based on a large dataset:
[math] R_2 = 1.1 \; (\eta_u + 0.5 \sqrt{S_w^2 + S_{ig}^2} \, ) , \qquad \xi \ge 0.3 , \qquad R_2= 0.043 \; \sqrt{HL} , \qquad \xi \lt 0.3 , \qquad (4) [/math]
where [math]\eta_u = 0.35 H \xi[/math] is the wave set-up, [math]S_w=0.75 H \xi[/math] is the swash uprush related to incident waves and [math]S_{ig}=0.06 \sqrt{HL}[/math] is the additional uprush related to infragravity waves. The factor 1.1 takes into account the non-Gaussian distribution of run-up events.
An empirical expression taking the medium sediment grain size [math]d_{50}[/math] [m] into account is[1][5],
[math]R = 0.4 \beta \,T \, \sqrt{gH} \, \exp(-10 \, d_{50}^{0.55}) \, . \qquad (5) [/math]
This formula is less suitable for dissipative beaches, where swash is dominated by infragravity waves. In this case a dependence on the square root of the beach (swash) slope should be preferred, e.g. [math]\,\eta_u = (0.2-0.3) \, \sqrt{\beta \, H_s \, L} \,[/math] [6][7]. Other studies find that run-up dominated by infragravity waves is generally small and increases with increasing wave height (approximately linear dependence[8][9]).
From an inventory of run-up formulas by Gomes da Silva et al. (2020[10]), it appears that for steep beaches ([math] \beta \gt 0.1[/math]) the run-up increases with increasing beach slope (approximately linear dependance[11]), while for gently sloping dissipative beaches ([math] \beta \lt 0.1[/math]) the dependence on beach slope is weak or absent[11][4]. Field observations[12] and numerical models[13] point to a dependence of infragravity swash on the frequency spread and the directional spread of incident waves. The largest infragravity swash has been observed for incident waves with a small directional spread and a large frequency spread.
Run-up on mixed sand-cobble beaches
Mixed sand-cobble beaches have a sandy upper shoreface and lower beach with typical slopes of 0.01-0.05 that dissipate most shortwave energy. The upper beach, in contrast, has a steep berm with a slope of 0.1-0.25 and is composed of coarse sediment. Due to cobble roughness, swash infiltration and reflection, backwash velocities are small. These beaches therefore tend to be stable, especially since cobble berms can also dynamically adjust to wave impacts (see Stability of rubble mound breakwaters and shore revetments). Sandy beach run-up formulas overpredict run-up on mixed beaches by ignoring the effects of increased percolation, reflection and roughness. Run-up observations on mixed sand-cobble beaches by Conlin et al. (2025[14]) were best simulated with the empirical expression
[math]R_2 = 1.3 \, (\eta_u + ½ S)\, , \quad \eta_u = 0.92 \, \beta \, H_s^{0.7}\, L^{0.3} \, , \quad S = 1.28 + 3 \, \beta \, H_s \, . \qquad (6)[/math]
Accuracy of run-up estimates
The accuracy (root-mean-square deviation) of run-up predictions using empirical formulas such as (1), (3) or (4) is usually not better than [math]0.25 \, R[/math].[3] The general applicability of empirical formulas for run-up prediction based on simple parametric representations of beach and shoreface is limited due to the influence of the more detailed characteristics of the local beach and shoreface bathymetry (e.g., beach permeability and groundwater level, curvature of the beach profile, crescentic bars, or swash acceleration along the horn of a beach cusp embayment[15]). The tide level may influence the wave run-up due to wave breaking on nearshore sandbars.[16] The applicability of empirical formulas for the wave set-up, which is a substantial component of the run-up, is limited for similar reasons. Accurate estimates of the wave run-up require in-situ observations or detailed numerical models. A more detailed discussion of wave run-up and backwash is given in the articles Swash and Swash zone dynamics. An analytical model estimate of the runup of monochromatic non-broken waves on a uniform sloping beach is discussed in the article Waves on a sloping bed.
Run-up saturation
The empirical formulas suggest that the run-up is an ever increasing function of the incident wave height. Field and laboratory observations show that this is not the case[17][18]. Several processes limit the uprush on the beach when incident waves are very high: breaking of short waves in the surf zone (leading to surf zone saturation), breaking of infragravity waves on the beach face and collision of the uprushing swash bore with the backwash of the preceding swash bore. The greatest wave run-up occurs for the highest waves that do not break, which are generally waves in the lower part of the wave frequency spectrum (long-period waves). Theoretical formulas for run-up saturation have the form (see appendix)
[math]R \lt R_s = A \, g \, \beta^2 \, T^2 \, . \qquad (7)[/math]
Using for the coefficient [math]A[/math] the value [math]A \approx 0.14[/math], reasonable agreement is found with field and laboratory observations. [18]
Appendix
For monochromatic frictionless waves, the ballistic model gives the run-up [math]R \approx 2 D_0[/math], where [math]D_0[/math] is the height of the wave collapsing on the beach (see Swash#Appendix: Ballistic swash model, Eq. A2). Non-breaking waves can reach greater height than breaking waves. In Waves on a sloping bed#Wave breaking (Eq. 30), a theoretical criterion for wave breaking is [math]\; D_0 \lt 2 g \beta^2 / \omega^2 = g \beta^2 T^2 / (2 \pi^2)[/math]. The highest run-up (saturation) is thus given by [math]\; R_s = g \beta^2 T^2 / \pi^2 .[/math]
Uprush is also limited if it collides with the preceding backwash. This happens when the swash period is longer than the period of the incident waves. Another criterion for maximum runup is therefore a shorter swash period than wave period. According to the ballistic model this condition is [math]\; R_s = g \beta^2 T^2 / 8 [/math] (see Swash#Swash saturation).
Related articles
References
- ↑ Jump up to: 1.0 1.1 Hunt, I.A. 1959. Design of seawalls and breakwaters. J. Waterw. Harbors Division ASCE 85: 123–152
- Jump up ↑ Holman, R.A. and Sallenger, A.H. 1985. Setup and swash on a natural beach. J. Geophys. Res. 90: 945–953
- ↑ Jump up to: 3.0 3.1 Atkinson, A.L., Power, H.E., Moura, T., Hammond, T., Callaghan, D.P. and Baldock, T.E. 2017. Assessment of runup predictions by empirical models on non-truncated beaches on the south-east Australian coast. Coast. Eng. 119: 15–31
- ↑ Jump up to: 4.0 4.1 4.2 Stockdon, H.F., Holman, R.A., Howd, P.A. and Sallenger, A.H. 2006. Empirical parameterization of setup, swash, and runup. Coast. Eng. 53: 573–588
- Jump up ↑ Sunamura,T. 2004. A predictive relationship for the spacing of beach cusps in nature. Coastal Engineering 51: 697-711
- Jump up ↑ Medellín, G., Brinkkemper, J.A., Torres-Freyermuth, A., Appendini, C.M., Mendoza, E.T. and Salles, P. 2016. Run-up parameterization and beach vulnerability assessment on a barrier island: a downscaling approach. Nat. Hazards Earth Syst. Sci. 16: 167–180
- Jump up ↑ Gomes da Silva, P., Medina, R., González, M. and Garnier, R. 2018. Infragravity swash parameterization on beaches: the role of the profile shape and the morphodynamic beach state. Coast. Eng. 136: 41–55
- Jump up ↑ Ruessink, B.G., Kleinhans, M.G. and Van Den Beukel, P.G.L. 1998. Observations of swash under highly dissipative conditions. J. Geophys. Res. 103: 3111–3118
- Jump up ↑ Ruggiero, P., Holman, R. A. and Beach, R. A. 2004. Wave run-up on a high-energy dissipative beach. J. Geophys. Res. 109, C06025, doi:10.1029/2003JC002160
- Jump up ↑ Gomes da Silva, P., Coco, G., Garnier, R. and Klein, A.H.F. 2020. On the prediction of runup, setup and swash on beaches. Earth-Science Reviews 204, 103148
- ↑ Jump up to: 11.0 11.1 Nielsen, P. and Hanslow, D.J. 1991. Wave runup distributions on natural beaches. J. Coast. Res. 7: 1139–1152
- Jump up ↑ Matsuba, Y. and Shimozono, T. 2021. Analysis of the contributing factors to infragravity swash based on long-term observations. Coastal Engineering 169, 103957
- Jump up ↑ Guza, R.T. and Feddersen, F. 2012. Effect of wave frequency and directional spread on shoreline runup. Geophys. Res. Lett. 39: 1–5
- Jump up ↑ Conlin, M.P., Wilson,G., Bond, H., Ardag, G. and Arnowil, C. 2025. Predicting wave runup on composite beaches. Coastal Engineering 199, 104743
- Jump up ↑ Kim, L.N., Brodie, K.L., Cohn, N.T., Giddings, S.N. and Merrifield, M. 2023. Observations of beach change and runup, and the performance of empirical runup parameterizations during large storm events. Coastal Engineering 184, 104357
- Jump up ↑ Guedes, R.M.C., Bryan, K.R., Coco, G. and Holman, R.A. 2011. The effects of tides on swash statistics on an intermediate beach. J. Geophys. Res. 116, C04008
- Jump up ↑ Guza, R.T., Thornton, E.B. and Holman, R.A. 1984. Swash on steep and shallow beaches. Proceedings of the 19th International Conference Coastal Engineering, ASCE, 1, pp. 708 – 723
- ↑ Jump up to: 18.0 18.1 Hughes, M.G., Baldock, T.E. and Aagaard, T. 2017. Swash saturation: is it universal and do we have an appropriate model? Coastal Dynamics Conf. 2017, Paper No.108, pp. 192-203
Please note that others may also have edited the contents of this article.
|