Bedforms and roughness

Definition of Bedforms: Bedforms are relief features generated by fluid flow over a bed consisting of movable sediments. This is the common definition for Bedforms, other definitions can be discussed in the article

Introduction

Many types of bed forms can be observed in nature. The bed form regimes for steady flow over a sand bed can be classified into (see Figure 1):

• lower transport regime with flat bed, ribbons and ridges, ripples, dunes and bars,
• transitional regime with washed-out dunes and sand waves,
• upper transport regime with flat mobile bed and sand waves (anti-dunes).

When the bed form crest is perpendicular (transverse) to the main flow direction, the bed forms are called transverse bed forms, such as ripples, dunes and anti-dunes. Ripples have a length scale much smaller than the water depth, whereas dunes have a length scale much larger than the water depth. The crest lines of the bed forms may be straight, sinuous, linguoid or lunate. Ripples and dunes travel downstream by erosion at the upstream face (stoss-side) and deposition at the downstream face (lee-side). Antidunes travel upstream by lee-side erosion and stoss-side deposition. Bed forms with their crest parallel to the flow are called longitudinal bed forms such as ribbons and ridges.

In the literature, various bed-form classification methods for sand beds are presented. The types of bed forms are described in terms of basic parameters (Froude number, suspension parameter, particle mobility parameter; dimensionless particle diameter).

A flat immobile bed may be observed just before the onset of particle motion, while a flat mobile bed will be present just beyond the onset of motion. The bed surface before the onset of motion may also be covered with relict bed forms generated during stages with larger velocities.

Figure 1: Bed forms in steady flows (rivers). From Simons and Richardson (1966).

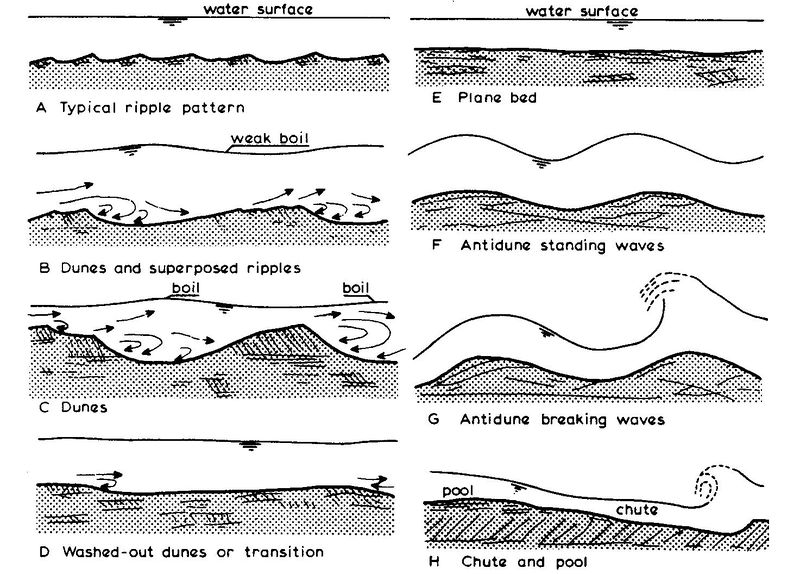
Bedforms in unidirectional and oscillating flow

The main focus of this article is on bedforms observed in rivers. Many of the processes that generate bedforms in unidirectional flow also generate bedforms in oscillatory flows. This holds for dunes and bars which have similar characteristics in rivers and estuaries. Bed ripples are an ubiquitous feature in rivers as well as in coastal environments. However, wave-generated ripples have specific characteristics and are therefore discussed in two separate articles, Wave ripples and Wave ripple formation. Empirical formulas of bedform characteristics and bed roughness are given in the Appendix, including wave-generated ripples. Formulas for estuaries are given in the article Bed roughness and friction factors in estuaries.

Current-generated ripples

Small-scale ribbon and ridge type bed forms parallel to the main flow direction have been observed in laboratory flumes and small natural channels, especially in case of fine sediments (grainsize [math]d_{50}[/math] typically smaller than 0.1 mm). They are probably generated by secondary flow phenomena and near-bed turbulence effects (burst-sweep cycle) in the lower and transitional flow regime. These bed forms are also known as parting lineations because of the streamwise ridges and hollows with a vertical scale equal to about 10 grain diameters. These bed forms are mostly found in fine sediments ([math]0.05 \lt d_{50} \lt 0.25 \; mm[/math]).

When the velocities are somewhat larger (10%-20%) than the critical velocity for initiation of motion and the median particle size is smaller than about 0.5 mm, small (mini) ripples are generated at the bed surface. Ripples that are developed during this stage remain small with a ripple length much smaller than the water depth. The characteristics of mini ripples are commonly assumed to be related to the turbulence characteristics near the bed (burst-sweep cycle). Current ripples have an asymmetric profile with a relatively steep downstream face (lee-side) and a relatively gentle upstream face (stoss-side). As the velocities near the bed become larger, the ripples become more irregular in shape, height and spacing yielding strongly three-dimensional ripples. In this case the variance of the ripple length and height becomes rather large. These ripples are known as lunate ripples when the ripple front has a concave shape in the current direction (crest is moving slower than wing tips) and are called linguoid ripples when the ripple front has a convex shape (crest is moving faster than wing tips). The largest ripples may have a length up to the water depth and are commonly called mega-ripples.

Dunes

Another typical bed form type of the lower regime is the dune-type bed form. Dunes have an asymmetrical (triangular) profile with a rather steep lee-side and a gentle stoss-side. A general feature of dune type bed forms is lee-side flow separation resulting in strong eddy motions downstream of the dune crest. The length of the dunes is strongly related to the water depth ([math]h[/math]) with values in the range of [math](3 - 15) h[/math], with [math](6 - 7) h[/math] as most usual value. Extremely large dunes with heights ([math]\Delta[/math]) of the order of 7 m and lengths ([math]\lambda[/math]) of the order of 500 m have been observed in the Rio Parana River (Argentina) at water depths of about 25 m, velocities of about 2 m/s and bed material sizes of about 0.3 mm. Field observations show that dunes can develop from an initial ripple field by a nonlinear process of pattern coarsening, i.e. successive stages of ripple merging and growth .

Sand bars

The largest bed forms in the lower regime are sand bars (such as alternate bars, side bars, point bars, braid bars and transverse bars), which usually are generated in areas with relatively large transverse flow components (bends, confluences, expansions). Alternate bars are features with their crests near alternate river bends. Braid bars actually are alluvial "islands" which separate the anabranches of braided streams. Numerous bars can be observed distributed over the cross-sections. These bars have a marked streamwise elongation.

Transitional regime

It is a well-known phenomenon that the bed forms generated at low velocities are washed out at high velocities. It is not clear, however, whether the disappearance of the bed forms is accomplished by a decrease of the bed form height, by an increase of the bed form length or both. Flume experiments with sediment material of about 0.45 mm show that the transition from the lower to the upper regime is effectuated by an increase of the bed form length and a simultaneous decrease of the bed form height. Ultimately, relatively long and smooth sand waves with a roughness equal to the grain roughness were generated .

In the transition regime the sediment particles will be transported mainly in suspension. This will have a strong effect on the bed form shape. The bed forms will become more symmetrical with relatively gentle lee-side slopes. Flow separation will occur less frequently and the effective bed roughness will approach to that of a plane bed. Large-scale bed forms with a relative height ([math]\Delta / h[/math]) of 0.1 to 0.2 and a relative length ([math]\lambda / h[/math]) of 5 to 15 were present in the Mississippi river at high velocities in the upper regime.

Antidunes

In the supercritical upper regime the bed form types will be plane bed and/or anti-dunes. The latter type of bed forms are sand waves with a nearly symmetrical shape in phase with the water surface waves. The anti-dunes do not exist as a continuous train of bed waves, but they gradually build up locally from a flat bed. Anti-dunes move upstream due to strong lee-side erosion and stoss-side deposition. Anti-dunes are bed forms with a length scale of less than 10 times the water depth. When the flow velocity further increases, finally a stage with chute and pools may be generated.

Formation of bedforms

Fig. 2. Schematic representation of bedform formation. Top: Steady flow from left to right. Two successive stages of bed perturbation development are brown and yellow, respectively. The dark-blue dotted line is a near-bed streamline. The dark-blue arrows are flow vectors. Middle: Steady flow from right to left. Under: Bedform formation in oscillating flow as linear superposition of the two steady flow situations.

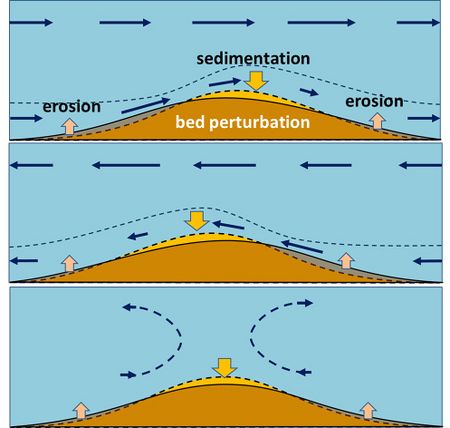
The formation of bedforms is related to the inherent instability of the sediment bed. It can be qualitatively understood as follows. A small perturbation of the sediment bed generates a perturbation of the flow pattern and associated zones of sediment transport convergence and divergence. The perturbation will grow if the sediment transport convergence zone comprises the crest region of the initial perturbation. The underlying cause is the short range of the additional turbulent friction and momentum dissipation produced by the bed perturbation. Consider, for example, a bed perturbation that restricts the flow depth (or the flow width) of a channel. While passing the perturbation crest, the flow accelerates. This will be the case for the flow zone most remote from the bed perturbation (near the surface or opposite the width restriction), which is hardly influenced by the additional frictional momentum dissipation generated by the perturbation. In contrast, the flow in the zone closest to the bed perturbation is strongly perturbed. After an initial acceleration on the upstream ramp, the flow slows down before passing the perturbation crest due to the increasing shear stress. This deceleration increases the internal velocity shear and thus counteracts the acceleration of the remote flow downstream of the bed perturbation. The flow deceleration close to the bed perturbation locally diminishes the sediment transport capacity. This implies sediment deposition, that leads to growth of the perturbation if it is assumed that most of the sediment load is transported close to the bed. The process is schematically illustrated in Fig. 2, for unidirectional flow and for oscillating flow.

Different types of bedforms can be generated through this mechanism, depending on their impact on the structure and intensity of turbulent fluid motions and momentum dissipation in steady flow, tidal flow or wave dominated flow. The type of perturbations with the highest initial growth rate will become the dominant bedform. Perturbations with small length scales produce the strongest turbulence and will thus be favoured. However, bedforms with small length scales are steep and therefore subject to avalanching. Due to this antagonistic mechanism, the fastest growing bedforms are not the steepest ones, but bedforms with intermediate length scales. These length scales can be estimated by performing a linear stability analysis, as explained in the articles Stability models and Wave ripple formation. In this way it is possible to understand the formation of almost all bedforms that are observed in sandy coastal environments. See also the articles Rhythmic shoreline features and Sand ridges in shelf seas.

Bed roughness

Nikuradse introduced the concept of an equivalent or effective sand roughness height, [math]k_s[/math], to simulate the roughness of arbitrary roughness elements of the bottom boundary. In case of a movable bed consisting of sediments the effective bed roughness [math]k_s[/math] mainly consists of grain roughness ([math]k'_s[/math]) generated by skin friction forces and of form roughness ([math]k''_s[/math]) generated by pressure forces acting on the bed forms. Similarly, a grain-related bed-shear stress ([math]\tau'_b[/math]) and a form-related bed-shear stress ([math]\tau''_b[/math]) can be defined. The effective bed roughness for a given bed material size is not constant but depends on the flow conditions. Analysis results of [math]k_s[/math]-values computed from Mississippi River data (USA) show that [math]k_s[/math] strongly decreases from about 0.5 m at low velocities (0.5 m/s) to about 0.001 m at high velocities (2 m/s), probably because the bed forms become more rounded or are washed out at high velocities.

The fundamental problem of bed roughness prediction is that the bed characteristics (bed forms) and hence the bed roughness depend on the main flow variables (depth, velocity) and sediment transport rate (sediment size). These hydraulic variables are, however, in turn strongly dependent on the bed configuration and its roughness.

A second problem is the almost continuous variation of the discharge during rising and falling stages. Under these conditions the bed form dimensions and hence the friction coefficient are not constant but vary with the flow conditions. Bedforms observed in the field are generally in a transient state.

A third problem is the influence of biota on bed friction. Micro-organisms living in and on the bed can stick sediments together and reduce bed friction by forming surface films (algae mats) and by excreting large organic molecules (EPS). Other organisms can enhance sediment mobility and bed friction through bioturbation. Bed friction can be strongly reduced by seagrass and seaweed. For a discussion of these various biotic factors, see Biogeomorphology of coastal systems.

Related articles

Bed roughness and friction factors in estuaries

Wave ripples

Stability models

Wave ripple formation

Rhythmic shoreline features

Sand ridges in shelf seas

Biogeomorphology of coastal systems

Appendix Empirical estimates of bedform characteristics and associated bed roughness

Several formulas have been derived from laboratory and field experiments for estimating bedform characteristics (especially height and wavelength). Bedforms influence currents and waves by enhancing shear stresses and associated frictional momentum dissipation in the turbulent boundary layer. Conversely, currents and waves are themselves the primary agents responsible for the emergence and development of these bedforms by inducing sediment transport. Spatial patterns in sediment transport are both cause of and caused by the development of bedforms due to the inherent instability of the sediment bed. Bedform characteristics depend on flow properties and flow properties depend on bedform characteristics. The empirical formulas presented in this appendix establish relationships between flow properties and bedform characteristics.

The case of currents only (no waves)

Steady or low-periodic water motions in coastal waters are turbulent over the whole water column. The shear stress [math]\tau_b[/math] is related to the depth-averaged current velocity [math]U[/math] through the friction coefficient [math]c_D[/math]:

[math]c_D = \Large\frac{\tau_b}{\rho U^2}\normalsize ,[/math]

where [math]\rho[/math] is the density of water. (Sometimes other friction factors are used, such as the Chezy coefficient, the Darcy-Weisbach friction factor or the Manning coefficient, see Bed roughness and friction factors in estuaries). The friction coefficient [math]c_D[/math] is related to the thickness [math]k_s[/math] of the near-bed roughness layer (the Nikuradse length) ,

[math]k_s = 12 h \exp(\large\frac{0.16}{\sqrt{c_D}}\normalsize) , [/math]

where [math]h[/math] is the total water depth.

For a flat sediment bed [math]k_s \approx (2-3) \, d_{50} ,[/math] where [math]d_{50}[/math] is the median sediment grainsize.

For a fully developed field of bottom ripples with flow-perpendicular straight crestlines the ripple height [math]\eta_r[/math] and ripple wavelength [math]\lambda_r[/math] can be estimated with empirical formulas established by Soulsby et al. (2012),

[math]\eta_r \approx 202 \, d_{50} \, d_*^{-0.554} , \quad \lambda_r \approx d_{50} \, (500 + 1881 \, d_*^{-1.5}) ,[/math]

where the dimensionless grainsize [math]d_*[/math] is defined as [math]d_* = d_{50} \, \big( \large\frac{g (s-1)}{\nu^2}\normalsize \big)^{1/3} \approx 25,000 \, d_{50}[/math], and where the relative sediment density [math]s = \rho_{sediment}/\rho \approx 1.65[/math] and the kinematic viscosity of water [math]\nu \approx 10^{-6} m^2/s[/math].

Flemming (1988) derived from field observations in rivers and estuaries the relationship [math]\quad \eta_r = 0.677 \, \lambda_r^{0.809} . [/math]

Lapotre et al. (2017) derived a relationship for the ripple wavelength based on field data and dimensional analysis, [math]\quad \lambda_r \approx 2504 \, d_{50}^{1/3} \Theta^{1/6} (\nu/u_*)^{2/3} ,[/math]

where [math]\Theta = \Large\frac{\tau_{b}}{g \rho (s-1) d_{50}}\normalsize[/math] is the Shields parameter, [math]\tau_b = \rho u_*^2[/math] is the bed shear stress and [math]u_*[/math] is the friction velocity

The roughness height [math]k_s[/math] according to van Rijn (2007) can be estimated from

[math]k_s = d_{50} \, \big[85 – 65 \, \tanh(0.015(\Psi-150)) \big] ,[/math]

where [math]\Psi = \Large\frac{U^2}{g (s-1)d_{50}}\normalsize[/math] is the mobility parameter.

Venditti and Bradley (2022) established empirical relations for the height and wavelength of dunes based on field observations, [math]\quad \log_{10}(\Large\frac{\eta_r}{h}\normalsize) = - 0.397\, \big[ \log_{10}(\Large\frac{\Theta}{\Theta_{cr}}\normalsize) -1.14 \big]^2 - 0.503, \quad \log_{10}(\Large\frac{\lambda_r}{h}\normalsize) = 0.098\, \big[ \log_{10}(\Large\frac{\Theta}{\Theta_{cr}}\normalsize) - 1.09 \big]^2 + 0.791 ,[/math]

where [math]\Theta_{cr} \approx 0.05 [/math] is the critical value of the Shields parameter for the onset of particle motion.

The case of waves only

The wave orbital motion along the bottom changes direction frequently. This hampers the development of the turbulent boundary layer. The height of the wave boundary layer [math]h_w[/math] is generally small, of the order of one to ten centimeters. It depends on the wave phase and is largest shortly before reversal of the orbital motion. For a rough sediment bed the height of the wave boundary layer depends on the height [math]k_s[/math] of the roughness elements, such as the median sediment grainsize [math]d_{50}[/math], the wave orbital excursion [math]2a[/math] and the ripple characteristics (height [math]\eta_r[/math] and wavelength [math]\lambda_r[/math]) for a rippled bed. The wave orbital excursion is related to the maximum wave orbital velocity [math]U_b[/math] at the top of the wave boundary layer, [math]a= U_b / \omega [/math], where [math]\omega=2 \pi / T[/math] is the wave angular frequency. The following empirical order-of-magnitude estimates have been derived based on laboratory and field experiments:

• For a smooth flat sediment bed [math]h_w \approx \sqrt {\nu T /\pi }[/math], where [math]T[/math] is the wave period
• For flat rough bed [math]k_s \approx (2-3) \, d_{50}[/math] and [math]\; h_w \approx 0.09 \, k_s (\large\frac{a}{k_s}\normalsize)^{0.82}[/math]
• For a stone-covered bed [math]\; h_w \approx 0.08 \, k_s \big[ 1 + (\large\frac{a}{k_s}\normalsize)^{0.82} \big] [/math]
• For a rippled bed [math]\; k_s \approx 8 \Large\frac{\eta_r^2}{\lambda_r}\normalsize + 170 \, d_{50} \sqrt{\Theta - \Theta_{cr}}[/math].

The symbols are defined as follows:

• [math]\Theta = \Large\frac{\tau_{bw}}{g \rho (s-1) d_{50}}\normalsize[/math] is the Shields parameter
• [math]\Theta_{cr} \approx 0.165\, (R_f+0.6)^{-0.8} + 0.045 \, \exp[-40 R_f^{-1.3}] [/math] is the critical value of the Shields parameter for the onset of particle motion
• [math]\tau_{bw}[/math] is the maximum wave-induced shear stress
• [math]R_f=\sqrt{\large\frac{f_w}{2}}\large\frac{d_{50} U_b}{\nu}\normalsize[/math] is the grain Reynolds number

Order-of-magnitude estimates are [math]\Theta_{cr} \approx 0.05 , \; k_s \approx 25 \Large\frac{\eta_r^2}{\lambda_r}\normalsize [/math].

Empirical formulas for the ripple height and wavelength of a fully developed wave ripple field (orbital ripples) were established by Nielsen (1981),

[math]\eta_r \approx a \, (0.275 - 0.022 \, \Psi^{0.5}) , \quad \lambda_r \approx a \, (2.2 - 0.345 \, \Psi^{0.34}) , \quad[/math] where [math]\Psi = \Large\frac{U_b^2}{g (s-1) d_{50}}\normalsize[/math] is the sediment mobility parameter.

A revised formula of the ripple wavelength based on a larger dataset is [math]\quad \lambda_r \approx a\, (1.97 - 0.44 \, \Psi^{0.21})[/math].

Other empirical formulas for the ripple height and wavelength were given

by Soulsby and Whitehouse (2005) [math]\quad \eta_r \approx 0.15 \, \lambda_r \big[1-\exp(-(5000 \large\frac{d_{50}}{a}\normalsize)^{3.5}) \big] , \quad \lambda_r \approx a \, \Big(1+0.00187 \large\frac{a}{d_{50}}\normalsize \big[1-\exp(-(5000 \large\frac{d_{50}}{a}\normalsize)^{-1.5}) \big] \Big)^{-1} ,[/math]

by Goldstein et al. (2013) [math]\quad \eta_r \approx 313 \, d_{50} \, \lambda_r , \quad \lambda_r \approx 2a \, (1.12 + 2180 \, d_{50})^{-1} ,[/math]

by Ruessink et al. (2015) [math]\quad \eta_r \approx 0.164 \, \lambda_r (1-\tanh(0.63 \Theta)) , \quad \lambda_r \approx 0.676 \, a \, \Theta^{-0.163} \quad[/math] for [math]d_{50}\gt 0.3 mm[/math].

The wavelength of orbital ripples has order of magnitude [math]\lambda_r \sim 1.35 \, a[/math] and the ripple height is about a factor 10 smaller.

The wave friction coefficient is defined as

[math]f_w = \Large\frac{2 \, \tau_{bw}}{\rho U_b^2}\normalsize .[/math]

Several empirical formulas have been established for the wave friction coefficient. Most of the formulas, valid for [math]a\gt 1.6 k_s[/math], are of the form

[math]f_w = c_1 \, \exp\big[c_2 \, (\large\frac{a}{k_s}\normalsize)^{c_3} \big],[/math]

with the following values: ([math]c_1 = 0.00251, c_2 = 4.57, c_3 = -0.19[/math]) , ([math]c_1=0.00123, c_2 = 5.5, c_3 = -0.16[/math]) , ([math]c_1 = 0.00184, c_2 = 5.5, c_3 = -0.2[/math]).

For smooth sediment beds the friction coefficient is related to the Reynolds number [math]Re=\Large\frac{a U_b}{\nu}\normalsize[/math] : [math]f_w \approx 2 \; Re^{- 0.5} [/math] in case of laminar flow and otherwise [math]f_w \approx 0.035 \, Re^{-0.16}[/math].

For beds covered with very large roughness elements ([math]a/k_s \sim O[1][/math]) the friction coefficient can be approximated by [math]\; f_w \approx 0.32 \, (a/k_s)^{-0.8}[/math] and for the opposite case [math]a \gt \gt k_s[/math] , [math]\; f_w \approx 0.04 \, (a/k_s)^{- 0.25}[/math].

References

The main authors of this article are Leo van Rijn and Job Dronkers Please note that others may also have edited the contents of this article. Citation: Leo van Rijn; Job Dronkers; (2023): Bedforms and roughness. Available from http://www.coastalwiki.org/wiki/Bedforms_and_roughness [accessed on 11-12-2024] For other articles by this author see Category:Articles by Leo van Rijn For other articles by this author see Category:Articles by Job Dronkers For an overview of contributions by this author see Special:Contributions/Leo van Rijn For an overview of contributions by this author see Special:Contributions/Dronkers J