Long-period lunar tides

From Coastal Wiki
Jump to: navigation, search

Tidal motion is the oscillation of ocean waters under the influence of the attractive gravitational forces of the Moon and the Sun. The frequencies of these oscillations are determined by the cycles in the motions of Moon, Sun and Earth. The amplitude of the tidal oscillations is very small compared to ocean depths. The ocean tidal oscillation in each point can therefore be represented by a linear superposition of sinusoidal tidal components with periods derived from the various astronomical cycles.

The Earth's and Moon's orbital motion are characterized by a limited number of fundamental periods:

  • [math]1/f_1=[/math] period of lunar declination = 27.321582 days
  • [math]1/f_2 =[/math] period of solar declination = 365.242199 days
  • [math]1/f_3=[/math] period of lunar perigee rotation = 8.847 years
  • [math]1/f_4 =[/math] period of lunar node precession = 18.613 years
  • [math]1/f_5=[/math] period of Earth's perihelion precession = 20940 years

(perigee = point in the Moon's elliptical orbit nearest to the earth, lunar nodes = points at which the Moon's orbit cuts the ecliptic, perihelion precession = drift of Earth's closest approach to the Sun)

This article considers the effect of the lunar node cycle and the lunar perigee cycle on ocean tides. The effect of shorter periods of the Earth's and Moon's orbital motion is discussed in the article Ocean and shelf tides.


The 18.6-year lunar node cycle

The lunar node cycle refers to the precession of the Moon's orbital plane, where the lunar nodes complete one revolution in about 18.6 years. The lunar node cycle produces an 18.6-year oscillation of the lunar orbital plane relative to the equatorial plane. The angle between lunar orbital plane and Earth's equatorial plane varies between 18 and 28 degrees.

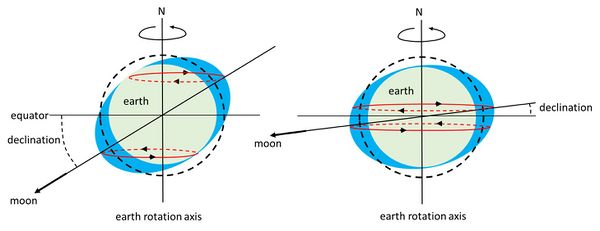
The primary driving force behind tidal motion on Earth is the gravitational pull exerted by the Moon. In a simplified model of an Earth without continents, the interaction between the Moon's gravity and the centrifugal acceleration resulting from Earth's orbit around the center of gravity of the Earth-Moon system creates two bulges on the ocean surface. One bulge is directed towards the Moon, while the other bulge is directed away from it (see Figure 1).

The Moon's orbital plane is inclined at an angle of approximately 5 degrees to the ecliptic plane, which is the plane of the Earth's orbit around the Sun. The Earth's equatorial plane is inclined at an angle of approximately 23 degrees to the ecliptic plane. In this article, the term lunar orbital inclination is used to designate the angle between the Moon's orbital plane and the Earth's equatorial plane. This angle is approximately equal to the maximum Moon declination reached during the monthly revolution around the Earth.

The lunar orbital inclination is not constant. It varies because the Moon's orbital plane slowly rotates with respect to the ecliptic plane. The line where the Moon's orbital plane intersects the ecliptic plane is called the line of lunar nodes. The two intersection points are the lunar nodes. The slow rotation of this line is called lunar nodal precession, or more precisely nodal regression. The lunar nodal cycle takes approximately 18.6 years to complete. Consequently, the lunar orbital inclination ranges between a maximum of approximately 28 degrees (23 + 5) and a minimum of approximately 18 degrees (23 - 5).


Fig. 1. Schematic equilibrium-tide illustration of how lunar declination affects the relative strength of semidiurnal and diurnal tidal forcing. The deformation is strongly exaggerated. In the real ocean, the response is modified by continents, bathymetry, Coriolis acceleration, resonance and dissipation. Left panel: For a large declination, a bulge passes through each point of the corresponding high latitudinal contour once a day, due to Earth’s rotation. Right panel: For a small declination, a bulge passes through each point of a low latitudinal contour twice a day. The rotation in the latitudinal planes is indicated in red.


Modulation of semidiurnal and diurnal tides

When the lunar orbital inclination is small, the semidiurnal tide is relatively strong. In the simplified equilibrium-tide representation, the two tidal bulges produced by the combined effect of lunar gravity and the centrifugal acceleration of the Earth-Moon system are then located close to the equatorial plane. Figure 1 schematically depicts this situation for the phase of the lunar nodal cycle when the lunar orbital inclination is small, with the Moon shown at its monthly phase of maximum declination. As a result, the tidal forcing at low and middle latitudes has a strong semidiurnal component, with two similar tidal pulses during each lunar day of about 24 hours and 50 minutes.

When the lunar orbital inclination is large, the tidal bulges in the equilibrium-tide representation are located farther from the equator during part of the Moon's monthly revolution. The two daily tidal pulses at a given latitude then become more unequal, producing a stronger diurnal component. This effect is greatest when the Moon reaches the maximum declination associated with this phase of the lunar nodal cycle, corresponding to maximum diurnal inequality of the tide.

Figure 1 gives a schematic representation of these two opposite phases of the lunar nodal cycle. The Moon declinations in the illustration are exaggerated for clarity.

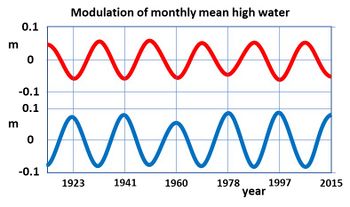
Consequently, the semidiurnal and diurnal tidal components reach their maximum strengths at opposite phases of the lunar nodal cycle, which lasts approximately 18.6 years. The time interval between these two phases is about 9.3 years, as shown in Figure 2.

Observed modulation of the semidiurnal and diurnal tidal amplitudes

Fig. 2. Representative modulation of monthly mean high water by the lunar nodal cycle. Blue: semidiurnal-dominated case, such as Brest (France). Red: diurnal-dominated case, such as Victoria (Canada). The curves illustrate the opposite nodal phases of semidiurnal and diurnal tidal regimes. Adapted from Peng et al. (2019[1]).


The idealized model of tide generation on an Earth without continents, the so-called equilibrium tide, does not accurately represent the actual tide generation process, which is influenced by the intricate topography of continental shelves. The strength of tidal motion in different locations on Earth is therefore determined not only by the intensity of the lunar tidal force but also by the resonance and dissipation processes of tidal motion resulting from topographic features.

The lunar node cycle modulates tidal constituents and therefore high-water levels. The resulting variation in monthly mean high water can range from only a few centimetres to about 30 cm, depending on location.[1] In relative terms, the diurnal components experience stronger modulation compared to the semidiurnal components. On average, the semidiurnal M2 tide is modulated by about 3-4%, while the diurnal tides O1 and K1 are modulated by approximately 11% and 19%, respectively.[2] As the semidiurnal tide tends to be larger than the diurnal tide in most ocean regions, the modulation exhibits a similar magnitude for both the semidiurnal and diurnal tidal components.

The character of the tide at a given location is often described by the tidal form factor, which is the ratio between the amplitudes of the main diurnal constituents and the main semidiurnal constituents:

[math]F = (K_1 + O_1)/(M_2 + S_2) \, , [/math],

where [math]K_1[/math] and [math]O_1[/math] are the main diurnal constituents and [math]M_2[/math] and [math]S_2[/math] the main semidiurnal constituents.

A small form factor indicates a mainly semidiurnal tide, whereas a large form factor indicates a mainly diurnal tide; intermediate values correspond to mixed tides. Because the lunar nodal cycle modulates diurnal and semidiurnal constituents with opposite phases (Fig. 2), the tidal form factor also varies over the 18.6-year cycle. In some mixed-tide regions, this variation is large enough for the local tide to shift temporarily from mixed semidiurnal toward more diurnal behavior, without any change in bathymetry or mean sea level.

The impact of the lunar node cycle on high-water levels is most pronounced in regions such as the English Channel, the Bristol Channel (both dominated by semidiurnal tides), and the Gulf of Tonkin (dominated by diurnal tides), where shifts between semidiurnal and mixed semidiurnal and between diurnal and mixed diurnal are observed over a period of 9.3 years.[3]

Impact of the lunar node cycle on coastal and marine processes

The lunar node cycle influences coastal processes that are regulated or modulated by tides. Because of the long periodicity, the influence of the lunar node cycle is easily confused with a change in long term trends. The influence of the lunar nodal tide on various coastal processes is discussed below.

Flooding risk

When heavy storms coincide with spring tidal high water, storm surge levels can be increased by a few centimeters up to a few tens of centimeters depending on the phase of the lunar orbital inclination. This should be reflected in the design conditions of coastal flood defenses in regions where the influence of the lunar node cycle on water level extremes is strong.[1] The nodal cycle is also important for high-tide or nuisance flooding. Where relative sea level rise has brought mean high water close to a flooding threshold, the nodal amplification of high waters can significantly increase the number of exceedance days during favorable phases of the cycle. This effect is predictable and should be included in short- to medium-term flood-frequency projections.[4][5]

Cooling and warming of ocean surface waters

An analysis of long-term records of sea surface temperatures in North American waters reveals a recurring cycle of approximately 19 years. Locations along the East Coast, which are primarily influenced by the semidiurnal tide, experience temperature minima during years of minimum lunar orbital inclination. In contrast, locations along the West Coast, dominated by diurnal tides, observe temperature minima during years of maximum lunar orbital inclination.[6]

This pattern can be attributed to nodal modulation of tidal amplitudes, which changes tidally induced mixing between warm surface waters and cooler deeper waters. Model studies support this mechanism and suggest weak but detectable regional climate signals, especially through changes in upper-ocean heat uptake. These effects are small compared with anthropogenic warming and major modes of internal climate variability, but they may contribute to decadal variability in some regions.[7][8]

Sediment deposition

Observations on the South American muddy coast, spanning 1500 km between the Amazon and Orinoco rivers, over the past twenty years reveal cycles of multiannual shoreline advance (progradation) and retreat (transgression). These cycles appear to be primarily influenced by the lunar 18.6-year node cycle. For this semidiurnal-dominated coast, the phase of minimum lunar orbital inclination corresponds to increased extreme high waters and enhanced shoreline retreat, whereas the opposite phase favors progradation. Conversely, during the phase of maximum lunar orbital inclination, the shoreline shows progradation. The long-term fluctuation of extreme tidal levels of about 6 cm induced by the lunar node cycle dominates over sea-level fluctuations from global warming or Niño-Niña events, and therefore provides a plausible explanation of the observed multiannual shoreline fluctuation.[9]

Tidal basin morphology

The strength of sediment transport in coastal environments depends on the intensity of the current, with the relationship typically following a power law between 3 and 5 (see Sediment transport formulas for the coastal environment). Where tidal-current amplitudes vary approximately in proportion to tidal range, a few percent nodal modulation of tidal range may produce a larger modulation of peak sediment transport, because many sediment transport formulae depend on current velocity to a power of about 3–5. Depending on the phase of the lunar node cycle, the maximum tide-induced sediment transport can be increased or decreased by up to 10%.

Observations have identified an 18.6-year cycle in the infill of the Wadden Sea tidal basins, indicating the influence of the lunar node cycle on sedimentation.[10] Similarly, the volume of the Humber estuary has shown an 18.6-year cycle.[11] Historic sedimentation records in shallow tidal seas, such as the Santa Barbara Basin, also exhibit cycles with a periodicity close to 18.6 years.[12] Furthermore, a dataset spanning 68 years has revealed that tidal channels in the Baie du Mont-Saint-Michel undergo alternating configurations over periods close to 18.6 years.[13]

Mangrove expansion and contraction

Observations indicate that the coverage of mangrove canopies along the Australian coastline increases or decreases based on the frequency and duration of inundation. Large parts of the gently sloping mudflats occupied by mangroves are only inundated when the tidal amplitude is sufficiently large. Since the 18.6-year lunar node cycle modulates the maximum tide levels, it thus influences the mangrove cover along the coast, in accordance with the observations.[14] Some coastal regions of Australia are dominated by semidiurnal tides and others by diurnal tides. The mangrove cover of these different regions shows contrasting multiannual fluctuations which are in line with the opposite phases of tidal amplitude modulation by the 18.6-year lunar node cycle.

El-Nino and Southern Oscillation

Some statistical studies have suggested a possible relation between the 18.6-year nodal cycle and ENSO variability, possibly through changes in tidal mixing. This link remains uncertain, and the nodal cycle should not be treated as a primary explanation for ENSO variability.[15]

Lunar nodal tide and mean sea level variation

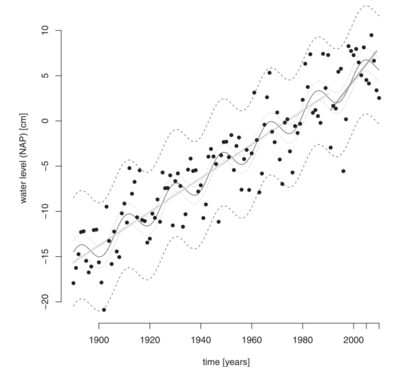
Fig. 3. Annual mean sea level averaged over six Dutch tidal stations (black dots). The modulation of the long-term linear trend represents the effect of the lunar nodal tide. The dotted lines represent confidence intervals. The linear fit at the end ignores the nodal tide and therefore overestimates the long-term trend. From Baart (2012[16]).

The gravitational pull exerted by the Moon on Earth, along with Earth's associated centrifugal acceleration, alters the shape of the ocean surface, as depicted in Fig. 1. This results in regions where the sea level is on average elevated (around the equator) and other regions where it is decreased (around the poles). The specific areas of increased and decreased ocean surface elevation fluctuate according to the Moon declination. This fluctuation gives rise to a sea level oscillation known as the lunar nodal tide, with a period of 18.6 years, see Appendix. It is important to note that this lunar nodal tide should not be confused with the previously discussed 18.6-year modulation observed in the semidiurnal and diurnal tides. The amplitude of the lunar nodal tide is generally less than 1 cm, which is an order of magnitude smaller than the effect of the nodal tide on the semidiurnal and diurnal tidal amplitudes and on Mean High High Water (MHHW).[17] Thus, the term 'lunar nodal tide' is best reserved for the long-period 18.6-year sea-level constituent, whereas 'nodal modulation' refers to the 18.6-year variation in the amplitudes and phases of ordinary tidal constituents.

Over records shorter than a few nodal cycles, the 18.6-year signal can bias estimates of local sea-level trends by a few tenths of a millimetre per year. This is small compared with present global mean sea-level rise, but large enough to affect detection of regional acceleration or trend breaks in tide-gauge records (Fig. 3).[16] It has also been suggested that in some regions (for example the western European coast) the 18.6-year modulation of the mean sea level is influenced by nodal modulation of tidal mixing in the upper ocean. This is due to the steric effect of the associated warming-cooling of the upper mixed layer of the adjacent ocean. It helps explain why observed tide-gauge nodal signals can differ from the theoretical equilibrium nodal tide.[18]

While there are arguments suggesting that the theoretical equilibrium tide should adequately represent the lunar nodal tide[19], analyses of tide gauge records question this assumption.[16][17][20] Woodworth (2012[21]) identified several potential causes for departures from equilibrium theory but determining the best method to correct for the effect of the nodal tide on the mean sea level is still a topic of ongoing discussion.

The 8.8-year lunar perigee cycle

The semidiurnal and diurnal tides are modulated also by another long-period lunar cycle, the 8.8-year cycle related to the elliptical orbit of the Moon. During each revolution of about 27 days the Moon is sometimes closer to the Earth and sometimes further away. The Moon's position closest to the Earth is called the perigee. The tidal forcing is strongest when the perigee is aligned with the Earth-Sun axis (leading to perigean spring tide), which occurs approximately twice a year. The strength of the semidiurnal tide also depends on the declination of the Sun, the angle of the Earth-Sun axis with the equatorial plane. The semidiurnal tide is strongest when the angle is zero, which occurs twice a year at equinox. Because the major axis of the Moon's elliptical orbit rotates 360o in about 8.8 years, the equinox and perigean spring-tide configuration recur in phase about every 4.4 years. Thus, the semidiurnal tide not only has a modulation of 18.6 years, but also a modulation of 4.4 years related to the Moon's 8.8-year perigee cycle.[22][23] The 4.4-year modulation of the semidiurnal tide is generally subordinate to the 18.6-year modulation. A 4.4-year modulation also exists in the diurnal tidal components (O1, K1). This small modulation is related to phase coincidence of the lunar perigee with the maximum declination of the sun.


Appendix

The equilibrium theory of tides, taking into account the solid Earth elastic response, self-attraction and mass conservation, gives the following estimate for amplitude of the 18.6-year nodal tide:[21] [math]\quad a \approx 7 \; | 3 \, \sin^2 \theta - 1|, \quad[/math] where [math]a[/math] is the amplitude in mm and [math]\theta[/math] the latitude in radians. The expression gives the amplitude; the sign of [math]3\sin^2\theta-1[/math] determines the phase. Hence low latitudes and high latitudes are out of phase, with a node at about 35° N/S. The amplitude is maximum at the poles and zero at 35 degrees N/S. High (> 35°) and low (< 35°) latitudes are out-of-phase; the phase at the equator corresponds to the phase of the nodal modulation of the semidiurnal tide.


Related articles

Ocean and shelf tides


Further reading

A physical and mathematical introduction useful for courses on tides and suited to researchers and engineers: Gerkema, T. 2019. An introduction to tides. Cambridge University Press, 222 pp.


References

  1. ↑ 1.0 1.1 1.2 Peng, D., Hill, E. M., Meltzner, A. J. and Switzer, A. D. 2019. Tide gauge records show that the 18.61‐year nodal tidal cycle can change high water levels by up to 30 cm. Journal of Geophysical Research: Oceans 124: 736–749
  2. ↑ Godin, G. 1972. The Analysis of Tides. University of Toronto Press, Ontario
  3. ↑ Pan, H., Wei, Y., Xu, T. and Wei, Z. 2024. A time-varying tidal form factor considering the 18.61-year nodal cycle. Estuarine, Coastal and Shelf Science 305, 108868
  4. ↑ Thompson, P.R., Widlansky, M.J., Hamlington, B.D., Merrifield, M.A., Marra, J.J., Mitchum, G.T. and Sweet, W. 2021. Rapid increases and extreme months in projections of United States high-tide flooding. Nature Climate Change 11: 584–590. https://doi.org/10.1038/s41558-021-01077-8
  5. ↑ Enríquez, A.R., Wahl, T., Baranes, H.E., Talke, S.A., Orton, P.M., Booth, J.F. and Haigh, I.D. 2022. Predictable changes in extreme sea levels and coastal flood risk due to long-term tidal cycles. Journal of Geophysical Research: Oceans 127(4): e2021JC018157. https://doi.org/10.1029/2021JC018157
  6. ↑ Loder, J. W. and Garrett, C. 1978. The 18.6-Year Cycle of Sea Surface Temperature in Shallow Seas. J. Geophys. Res. 83: 1967-1970
  7. ↑ Joshi, M., Hall, R., Stevens, D. and Hawkins, E. 2022. The modelled climatic response to the 18.6-year lunar node cycle and its role in decadal temperature trends. Earth Syst. Dynam. 14: 443–455
  8. ↑ Loder, J. W. and Garrett, C. 1978. The 18.6-Year Cycle of Sea Surface Temperature in Shallow Seas. J. Geophys. Res. 83: 1967-1970
  9. ↑ Gratiot, N., Anthony, E. J., Gardel, A., Gaucherel, C., Proisy, C. and Wells, J. 2008. Significant contribution of the 18.6 year tidal cycle to regional coastal changes. Nature Geoscience 1: 169–172
  10. ↑ Oost, A.P., de Haas, H., Ijnsen, F., van den Boogert, J.M. and de Boer, P.L. 1993. The 18,6 yr nodal cycle and its impact on tidal sedimentation. Sedimentary Geology 87: 1–11
  11. ↑ Wang, Z.B. and Townend, I.H. 2012. Influence of the nodal tide on the morphological response of estuaries. Marine Geology 291-294: 73–82
  12. ↑ Berger, W., Schimmelmann, A. and Lange, C. 2004. Tidal cycles in the sediments of Santa Barbara Basin. Geology 32: 329–332
  13. ↑ Levoy, F., Anthony, E.J., Dronkers, J., Monfort, O., Izabel, G. and Larsonneur, C. 2017. Influence of the 18.6-year lunar nodal tidal cycle on tidal flats: Mont-Saint-Michel Bay, France. Marine Geology 387: 108-113
  14. ↑ Saintilan, N., Lymburner, L., Wen, L., Haigh, I.D., Ai, E., Kelleway, J.L., Rogers, K., Pham, T.D. and Lucas, L. 2022. The lunar nodal cycle controls mangrove canopy cover on the Australian continent. Science Advances 8, eabo6602
  15. ↑ Yasuda, I. 2018. Impact of the astronomical lunar 18.6-yr tidal cycle on El-Niño and Southern Oscillation. Sci. Rep. 8, 15206
  16. ↑ 16.0 16.1 16.2 Baart, F., van Gelder, P. H. A. J. M., de Ronde, J., van Koningsveld, M. and Wouters C. 2012. The Effect of the 18.6-Year Lunar Nodal Cycle on Regional Sea-Level Rise Estimates, J. Coast. Res. 28: 511-516
  17. ↑ 17.0 17.1 Cherniawsky, J. Y., Foreman, M. G., Kang, S. K., Scharroo, R. and Eert, A. J. 2010. 18.6‐year lunar nodal tides from altimeter data. Continental Shelf Research 30: 575–587
  18. ↑ Bult, S.V., Le Bars, D., Haigh, I.D. and Gerkema, T. 2024. The effect of the 18.6-year lunar nodal cycle on steric sea level changes. Geophysical Research Letters 51(8): e2023GL106563
  19. ↑ Proudman, J. 1960. The condition that a long-period tide shall follow the equilibrium law. Geophysical Journal of the Royal Astronomical Society 3: 244–249
  20. ↑ Hagen, R., Plüß, A., Jänicke, L., Freund, J., Jensen, J. and Kösters, F. 2021. A combined modeling and measurement approach to assess the nodal tide modulation in the North Sea. Journal of Geophysical Research Oceans 126, e2020JC016364
  21. ↑ 21.0 21.1 Woodworth, P. L. 2012. A note on the nodal tide in sea level records. Journal of Coastal Research 28: 316-323
  22. ↑ Ray, R. D. and Merrifield, M. A. 2019. The semiannual and 4.4-year modulations of extreme high tides. Journal of Geophysical Research: Oceans 124: 5907–5922
  23. ↑ Haigh, I., Elliot, M. and Pattiaratchi, C. 2011. Global influence of the 18.6-year nodal cycle and 8.85-year cycle of lunar perigee on high tide levels. Journal of Geophysical Research 116, C06025

<ref

The main authors of this article are Job Dronkers and Fedor Baart
Please note that others may also have edited the contents of this article.
Citation: Job Dronkers; Fedor Baart; (2026): Long-period lunar tides. Available from http://www.coastalwiki.org/wiki/Long-period_lunar_tides [accessed on 2-07-2026]
Retrieved from "https://www.coastalwiki.org/w/index.php?title=Long-period_lunar_tides&oldid=82153"