Seawater density
Contents
[hide]Introduction
Density gradients play a prominent role in marine hydrodynamics. The large-scale long-term mean currents in the ocean and in estuaries largely depend on the density differences between water masses. The same holds for the small-scale turbulent velocity fluctuations that are responsible for mixing processes in oceans, shelf seas and estuaries. Knowledge of the seawater density is therefore prerequisite for understanding and modelling marine processes.
The seawater density [math]\rho[/math] in the ocean mainly depends on salinity [math]S[/math], temperature [math]T[/math] and pressure [math]p[/math]. Pressure only plays a role at depths much greater than those of coastal waters. Because of the primary focus of the Coastal Wiki on coastal waters, the dependency on pressure will be ignored in this article.
Salinity-temperature dependence of seawater density
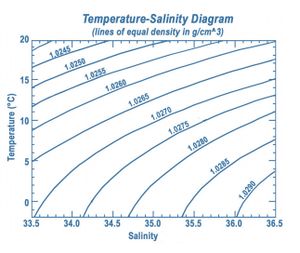
An accurate empirical expression for the density-salinity-temperature relationship for coastal waters has been derived by Fonofoff and Millard (1983[1]). This expression holds for the temperature range [math] 0\lt T\lt 40 \;[/math] oC, the salinity range [math] 0 \le S\lt 42 [/math] and for pressures lower than [math]p\lt 10[/math] bar. [math]S[/math] is the practical salinity.
[math]\rho = \rho_0 + (0.8245-4.1\, 10^{-3} \,T+7.64\, 10^{-5} \,T^2-8.25\, 10^{-7} \,T^3+5.4\, 10^{-9} \,T^4)\times S \\ +(-5.7\, 10^{-3} \,+10^{-4} \,T-1.65\, 10^{-6} \,T^2)\times S^{3/2} + 4.8\, 10^{-4}\,S^2 , \qquad (1)[/math]
[math]\rho_0 =999.84+6.8 \, 10^{-2} \, T - 9.1\, 10^{-3} \, T^2+10^{-4} \, T^3-1.1 \, 10^{-6} \, T^4+6.5\, 10^{-9} \, T^5 . \qquad (2)[/math]
The density-salinity-temperature relationship is plotted in Fig. 1. More precise relationships have been established since; these relationships also include pressure effects[2][3]. However, for coastal waters the increased accuracy is usually not required.
A simpler approximation of equations (1,2) with accuracy better than 1 ‰ is
[math]\rho = 999.1+ 0.77 \, (S-\Large\frac{T-15}{5.13}-\frac{(T-15)^2}{128}\normalsize) . \qquad (3)[/math]
In turbid coastal waters, the suspended sediment concentration in g / kg has to be added in this expression.
Processes induced by density differences
The density decreases with increasing temperature and increases with increasing salinity. According to Eq. (3), an increase of 1 g / kg in salinity has about the same effect on the seawater density as a decrease of 4-5 oC in temperature. Warmer water tends to stay near the surface and saltier water near the bottom. However, warm salty water can sink to the bottom whereas cold but less salty water can rise to the surface. The balance between small changes in salinity and temperature has great importance for the ocean circulation.
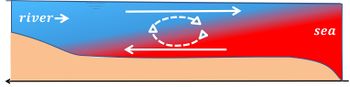
In estuaries the effect of the salinity gradient is generally much greater than the effect of the temperature gradient, producing the well-known estuarine circulation driven by a density gradient along the estuarine axis, see Fig. 2.
A body of water is referred to as 'stable' when the density decreases from bottom to surface. This is the usual situation, also in cases where strong mixing occurs over the water column. Mixing is mainly caused by turbulence, which is generated by frictional shear stresses at the bottom and the water surface. When the production of turbulent energy in an estuary decreases, inflowing river water and seawater will be less mixed: vertical density differences force the water column into a more stratified state, with the vertical density difference concentrated around a transition layer, the so-called pycnocline, see Fig. 3.
This is a nonlinear process as a result of the hydrostatic pressure differences (buoyancy principle of Archimedes) that dampen turbulent fluid fluctuations around the pycnocline; the turbulent energy production needed for mixing increases as the stratification increases. (Note: water and salt cannot spontaneously unmix from an existing solution, this would be contrary to the second law of thermodynamics. On the other hand, a suspension of fine sediment can unmix by settling.)
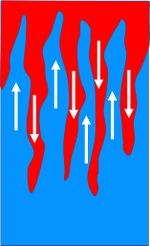
When saltier water is advected over less salty water by wind-driven or tide-driven currents, the water column becomes unstable. The saltier surface water will sink trough the less salty bottom. This process is called convective overturning and contributes to the mixing of water masses of different salinity. A similar process occurs when surface water is heated by the sun and increases its salinity through evaporation. The density of the warm salty surface water may then exceed the density of the underlying layer of cooler water, in which case the warm salty surface water will sink through this cooler layer. The downward motion is accelerated, because the salty surface water is cooled while sinking. This leads to the formation of so-called 'salt fingers', see Fig. 4. This overturning motion is not only important for the large scale ocean circulation, but it also causes upwelling of nutrient-rich water from the bottom layer and thus stimulates primary production on which the ocean food web depends.
Related articles
- Salinity
- Ocean circulation
- Thermohaline circulation of the oceans
- Estuarine circulation
- Salt wedge estuaries
References
- Jump up ↑ Fofonoff, N.P. and Millard, R.C. 1983. Algorithms for computation of fundamental properties of seawater. Unesco technical papers in marine science 44
- Jump up ↑ IOC, SCOR and IAPSO 2010. The international thermodynamic equation of seawater – 2010: Calculation and use of thermodynamic properties. Intergovernmental Oceanographic Commission, Manuals and Guides No. 56, UNESCO, 196 pp
- Jump up ↑ Schmidt, H., Seitz, S., Hassel, E and Wolf, H. 2018. The density–salinity relation of standard seawater. Ocean Sci.: 14, 15–40
Please note that others may also have edited the contents of this article.
|