Difference between revisions of "Wave-dominated river deltas"

From Coastal Wiki
Jump to: navigation, search
 
(4 intermediate revisions by 2 users not shown)
Line 34: Line 34:
 
'''What makes a delta wave-dominated?'''
 
'''What makes a delta wave-dominated?'''
  
−
Nienhuis <ref name="Nien15">Nienhuis, J. H., A. D. Ashton, and L. Giosan (2015), What makes a delta wave-dominated?, Geology, 43(6), 511–514, doi:10.1130/G36518.1. </ref> quantified wave-dominance by comparing the fluvial sand supply (Qriver in kg/s) to the ability of waves to move [[sediment]] from the [[river]] mouth (Qwave in kg/s). They defined a ratio R:
+
Nienhuis <ref name="Nien15">Nienhuis, J. H., A. D. Ashton, and L. Giosan (2015), What makes a delta wave-dominated?, Geology, 43(6), 511–514, doi:10.1130/G36518.1. </ref> quantified wave-dominance by comparing the fluvial sand supply (<math>Q_{river}</math> in [kg/s]) to the ability of waves to move sediment from the river mouth (<math>Q_{wave}</math> in [kg/s]). They defined a ratio <math>R</math>:
  
−
<math> R = \frac{Q_{river}}{Q_{wave}} </math>.
+
<math> R = \Large\frac{Q_{river}}{Q_{wave}}\normalsize </math>.
  
−
For a simple case where all [[waves]] approach shore normal, Qwave (in kg/s) can be approximated by,
+
For a simple case where all [[waves]] approach shore normal, <math>Q_{wave}</math> (in [kg/s]) can be approximated by,
  
 
<math> Q_{wave} = 90 \cdot H_s^{\tfrac{12}{5}} \cdot T_p^{\tfrac{1}{5}}</math>,
 
<math> Q_{wave} = 90 \cdot H_s^{\tfrac{12}{5}} \cdot T_p^{\tfrac{1}{5}}</math>,
  
−
where Hs is the significant waveheight (m) and Tp is the wave period (s).  
+
where <math>H_s</math> is the significant waveheight [m] and <math>T_p</math> is the wave period [s].  
 +
 
 +
If <math>R=0</math>, the river delta will be maximally wave dominated. For <math>R</math> approaching unity, the flanks of the wave-dominated delta will become increasingly pointy. If <math>R>1</math>, the river delta is expected to be river-dominated.
 +
 
 +
 
 +
[[Image:3.R WorldDeltas logaxis2-01.png|thumb|center|800px|River-dominance Ratio for several deltas]]
  
−
If R=0, the river delta will be maximally wave dominated. For R approaching unity, the flanks of the wave-dominated delta will become increasingly pointy. If R>1, the river delta is expected to be river-dominated.
 
  
 
==Changes to wave dominated deltas==
 
==Changes to wave dominated deltas==
Line 65: Line 69:
 
|AuthorFullName=Jaap Nienhuis
 
|AuthorFullName=Jaap Nienhuis
 
|AuthorName= Jaap Nienhuis}}
 
|AuthorName= Jaap Nienhuis}}
−
[[Category:Coastal processes, interactions and resources]]
+
 
−
[[Category:Geomorphological processes and natural coastal features]]  
+
[[Category:Physical coastal and marine processes]]
 
[[Category:Estuaries and tidal rivers]]
 
[[Category:Estuaries and tidal rivers]]
−
[[Category:Hydrodynamics]]  
+
[[Category:Sediment]]
−
[[Category:Land and ocean interactions]]
+
[[Category:Morphodynamics]]
−
[[Category:Hydrological processes and water]]
 

Latest revision as of 20:33, 3 July 2020

Definition of River delta:
A river delta is an accumulation of fluvially derived sediment that forms around the river mouth, where river water slows down as it enters a standing body of water [1].
This is the common definition for River delta, other definitions can be discussed in the article


Introduction

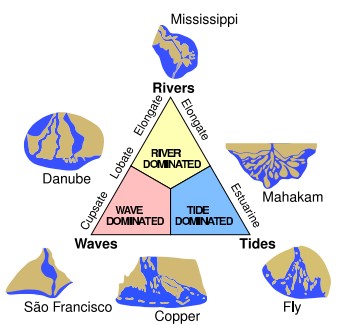
Figure 1: The ternary diagram of delta morphology [see [2]] showing wave dominated deltas in the lower left corner. Image [3]. Copyright Proceedings for the National Academy of Sciences.

The shape of a river delta, the delta morphology, depends on many factors such as waves, tides, sediment size and volumes, but also the space in which the delta grows [4]. In 1975, Galloway proposed that a characteristic delta morphology is associated with a particular combination of wave, tidal and fluvial factors, and developed a ternary diagram of delta morphology [2], see Fig. 1.

Wave-dominated deltas, such as the Nile Delta or the St. George lobe of the Danube Delta, are deltas where waves are the dominant factor shaping the fluvial sediment [5] . Compared to tidal and river-dominated deltas, wave dominated deltas often have smooth coastlines, and few (~1) distributary channels [2] (Fig. 2). In a recent study, Nienhuis [6] found that deltas attain a wave dominated shape when the coarse-grained fluvial sediment flux supplied to the river mouth is less than the maximum amount waves can transport away via alongshore sediment transport along both flanks of the delta. Deltas with a mixed influence of waves and tides [e.g., Niger, Mekong, [7] [8] often have multiple distributary channels with beach ridges on the islands in between channels.

Formation of wave-dominated river deltas

Figure 2: The Rio Grijalva in Mexico, highlighting an active wave-dominated delta and an abandoned wave-dominated delta revealed by the truncation of its beach ridges. Image NASA.

Waves affect the depositional patterns of sediment close to the river mouth. River mouth bars can form during floods, but in high wave energy environments waves will disperse the sediment alongshore and nourish the beaches adjacent to the river mouth [9] and the lower shoreface [10]. The short lifespan of river mouth bars in wave dominated environments results in the often few distributary channels of waves-dominated deltas, compared to deltas in low wave energy environments [11]. The continued progradation of wave dominated deltas can be recognized by beach ridges that leave traces of older shorelines [12] (Fig. 2).

Waves are an efficient sediment sorter, and move fine-grained sediment offshore while coarser grained sediment is left behind in the nearshore environment [13]. Wave dominated deltas are therefore often coarser than tide- or river dominated deltas [14]. River mouths not only distribute sediment to the coastal delta environment, they can also modify the transport of nearshore sediment fluxes [15]. One example is when river mouth currents (partially) block littoral sediment from bypassing the river mouth from the updrift to the downdrift coast [16] [17]. This effect is called the hydraulic groin effect, as it can result in the accumulation of sediment updrift of the river mouth, and erosion downdrift of the river mouth [16].

Cross-shore shape

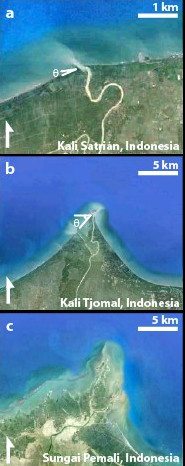
Figure 3: Three deltas with varying wave influence. (A) a strongly wave-dominated delta with a relatively flat cuspate shape. (B) A weakly wave-dominated delta with a more pointy cuspate shape, and (C), a river-dominated delta [after (Nienhuis 2015) [6].].

Waves affect the cross-shore profiles of river deltas. Waves generally steepen the shoreface [18] and transport fine-grained sediment further offshore. River dominated deltas, where waves are less important, frequently have mouth bar sands and fine-grained sediments up to mean sea level, whereas sediment cores of wave dominated deltas frequently show the coarsening upward sequence typical of wave influenced shorelines [19].

Plan-view shape

The plan-view shape of wave-dominated deltas is characterized by smooth shoreline shapes, and generally single threaded channels. The cuspate shape (Fig. 2) of wave dominated deltas tends to be symmetrical when waves approach perpendicular to the non-deltaic reference coastline [20]. Asymmetrical deltas can form in cases where the dominant wave approach is oblique [21]. Asymmetry is often manifested in the different delta sediments between the “updrift” delta flank facing the waves, and the “downdrift” delta flank that is more sheltered from the waves. Asymmetry around the river mouth can cause finer grained sediments to be trapped in the sheltered environment behind the river mouth bar. Additionally, when waves approach at an angle there can be a significant sediment supply from the beaches updrift of the delta that are not associated with the deltaic river mouth, but for example originate from a previously active delta lobe [e.g. Danube Delta [21]]. Recent model simulations also suggested that asymmetric wave approach can also cause deltaic channels to either prograde into or away from the direction of wave approach, depending on alongshore sediment bypassing, the directional wave climate, and fluvial sediment supply [22].

A study of delta on Java, Indonesia, showed that the pointiness of the cuspate shape reflects the degree of wave-dominance [6]. The most wave-dominated delta is a straight shoreline (no pointiness, e.g. Senegal), with high energy waves and relatively low fluvial sediment supply. Because alongshore sediment transport is dependent on the relative angle between shoreline and the offshore wave approach, and because the maximum alongshore sediment transport occurs when the waves approach the shoreline at ~45 degrees [23], the maximum pointiness a delta can attain is when the flanks are oriented at about 45 degrees (Fig. 4b). However, waves often approach form different angles, such that the maximum potential alongshore transport is lower, and the maximum pointiness is also lower than 45 degrees [6].

What makes a delta wave-dominated?

Nienhuis [6] quantified wave-dominance by comparing the fluvial sand supply ([math]Q_{river}[/math] in [kg/s]) to the ability of waves to move sediment from the river mouth ([math]Q_{wave}[/math] in [kg/s]). They defined a ratio [math]R[/math]:

[math] R = \Large\frac{Q_{river}}{Q_{wave}}\normalsize [/math].

For a simple case where all waves approach shore normal, [math]Q_{wave}[/math] (in [kg/s]) can be approximated by,

[math] Q_{wave} = 90 \cdot H_s^{\tfrac{12}{5}} \cdot T_p^{\tfrac{1}{5}}[/math],

where [math]H_s[/math] is the significant waveheight [m] and [math]T_p[/math] is the wave period [s].

If [math]R=0[/math], the river delta will be maximally wave dominated. For [math]R[/math] approaching unity, the flanks of the wave-dominated delta will become increasingly pointy. If [math]R\gt 1[/math], the river delta is expected to be river-dominated.


River-dominance Ratio for several deltas


Changes to wave dominated deltas

Because delta morphology is dependent on factors such as waves, tides, sediment supply, and sea level, changes to these factors often result in changes to delta morphology. One example is a delta avulsion, where an upstream change in the course of the river causes a rapid decrease in fluvial discharge and sediment flux to the previously active river mouth [24]. River dams are another example that also often result in a rapid decrease in the fluvial sediment supplied to the river mouth, either because of sediment blocking by the dam, or because of changes to the hydrograph that result in decreased downstream floods [25]. If the balance between the wave-dominated delta shape and the fluvial sediment flux is disturbed, a new “equilibrium” morphology will develop that will be more strongly wave-dominated in the case of fluvial sediment supply decreases. Delta sediment will be reworked by waves into spits [e.g., Ebro [26]] and barrier islands [e.g., Mississippi delta [24]].


References

  1. ↑ Credner, G. R. (1878), Die Deltas, edited by A. Peterman, Justus Perthes, Gotha
  2. ↑ 2.0 2.1 2.2 Galloway, W. D. (1975), Process Framework for describing the morphologic and stratigraphic evolution of deltaic depositional systems, in Deltas, Models for Exploration, edited by M. L. Broussard, pp. 86–98, Houston Geological Society, Houston, TX. Cite error: Invalid <ref> tag; name "Gal75" defined multiple times with different content Cite error: Invalid <ref> tag; name "Gal75" defined multiple times with different content
  3. ↑ Seybold, H., J. S. Andrade, and H. J. Herrmann (2007), Modeling river delta formation., Proc. Natl. Acad. Sci. U. S. A., 104, 16804–16809, doi:10.1073/pnas.0705265104.
  4. ↑ Coleman, J. M. (1981), Deltas. Processes of Deposition and Models for Exploration, 2nd ed., Burgess Publishing Company.
  5. ↑ Anthony, E. J. (2015), Wave influence in the construction, shaping and destruction of river deltas: A review, Mar. Geol., 361, 53–78, doi:10.1016/j.margeo.2014.12.004.
  6. ↑ 6.0 6.1 6.2 6.3 6.4 Nienhuis, J. H., A. D. Ashton, and L. Giosan (2015), What makes a delta wave-dominated?, Geology, 43(6), 511–514, doi:10.1130/G36518.1.
  7. ↑ Mcmanus, J. (2002), Deltaic responses to changes in river regimes, Mar. Chem., 155–170.
  8. ↑ Tamura, T., Y. Saito, V. L. Nguyen, T. K. O. Ta, M. D. Bateman, D. Matsumoto, and S. Yamashita (2012), Origin and evolution of interdistributary delta plains; insights from Mekong River delta, Geology, 40(4), 303–306, doi:10.1130/G32717.1.
  9. ↑ Limber, P. W., K. B. Patsch, and G. B. Griggs (2008), Coastal Sediment Budgets and the Littoral Cutoff Diameter: A Grain Size Threshold for Quantifying Active Sediment Inputs, J. Coast. Res., 2, 122–133, doi:10.2112/06-0675.1.
  10. ↑ Warrick, J. A., and P. L. Barnard (2012), The offshore export of sand during exceptional discharge from California rivers, Geology, 40(9), 787–790, doi:10.1130/G33115.1.
  11. ↑ Jerolmack, D. J., and J. B. Swenson (2007), Scaling relationships and evolution of distributary networks on wave-influenced deltas, Geophys. Res. Lett., 34(23), L23402, doi:10.1029/2007gl031823.
  12. ↑ Otvos, E. G. (2000), Beach ridges — definitions and significance, Geomorphology, 32(1-2), 83–108, doi:10.1016/S0169-555X(99)00075-6.
  13. ↑ Friedman, G. M. (1967), Dynamic processes and statistical parameters compared for size frequency distribution of beach and river sands, J. Sediment. Res., 37(2), 327–354, doi:10.1306/74D716CC-2B21-11D7-8648000102C1865D.
  14. ↑ Orton, G. J., and H. G. Reading (1993), Variability of deltaic processes in terms of sediment supply, with particular emphasis on grain size, Sedimentology, 40(3), 475–512, doi:10.1111/j.1365-3091.1993.tb01347.x.
  15. ↑ Zenkovich, V. P. (1967), Processes of Coastal Development, 1st ed., edited by J. A. Steers, Oliver & Boyd, Edinburgh.
  16. ↑ 16.0 16.1 Dominguez, J. M. L. (1996), The Sao Francisco strandplain: a paradigm for wave-dominated deltas?, Geol. Soc. London, Spec. Publ., 117(1), 217–231, doi:10.1144/GSL.SP.1996.117.01.13.
  17. ↑ Nienhuis, J. H., A. D. Ashton, W. Nardin, S. Fagherazzi, and L. Giosan (2016a), Alongshore sediment bypassing as a control on river mouth morphodynamics, J. Geophys. Res. Earth Surf., 121(4), 664–683, doi:10.1002/2015JF003780.
  18. ↑ Wright, L. D., and J. M. Coleman (1973), Variations in morphology of major river deltas as functions on ocean wave and river discharge regimes, Am. Assoc. Pet. Geol. Bull., 57(2), 370–398.
  19. ↑ Bhattacharya, J., and R. G. Walker (1991), River- and wave-dominated depositional systems of the Upper Cretaceous Dunvegan Formation, northwestern Alberta, Bull. Can. Pet. Geol., 39(2), 165–191.
  20. ↑ Komar, P. D. (1973), Computer models of delta growth due to sediment input from rivers and longshore transport, Bull. Geol. Soc. Am., 84(7), 2217–2226, doi:10.1130/0016-7606(1973)84<2217:CMODGD>2.0.CO;2.
  21. ↑ 21.0 21.1 Bhattacharya, J. P., and L. Giosan (2003), Wave-influenced deltas: geomorphological implications for facies reconstruction, Sedimentology, 50(1), 187–210, doi:10.1046/j.1365-3091.2003.00545.x.
  22. ↑ Nienhuis, J. H., A. D. Ashton, and L. Giosan (2016b), Littoral Steering of Deltaic Channels, Earth Planet. Sci. Lett., doi:10.1016/j.epsl.2016.08.018.
  23. ↑ Ashton, A. D., A. B. Murray, and O. Arnoult (2001), Formation of coastline features by large-scale instabilities induced by high-angle waves, Nature, 414(6861), 296–300, doi:10.1038/35104541.
  24. ↑ 24.0 24.1 Roberts, H. H. (1997), Dynamic changes of the Holocene Mississippi River delta plain: The delta cycle, J. Coast. Res., 13(3), 605–627.
  25. ↑ Milliman, J. D., K. L. Farnsworth, P. D. Jones, K. H. Xu, and L. C. Smith (2008), Climatic and anthropogenic factors affecting river discharge to the global ocean, 1951-2000, Glob. Planet. Change, 62(3-4), 187–194, doi:Doi 10.1016/J.Gloplacha.2008.03.001.
  26. ↑ Canicio, A., and C. Ibanez (1999), The Holocene Evolution of the Ebro Delta Catalonia, Spain, Acta Geogr. Sin., 54(5), 462–469.


Related articles

Morphology of estuaries


The main author of this article is Jaap Nienhuis
Please note that others may also have edited the contents of this article.
Citation: Jaap Nienhuis (2020): Wave-dominated river deltas. Available from http://www.coastalwiki.org/wiki/Wave-dominated_river_deltas [accessed on 22-11-2024]
Retrieved from "https://www.coastalwiki.org/w/index.php?title=Wave-dominated_river_deltas&oldid=76935"