Coastal Hydrodynamics And Transport Processes

The hydrodynamic conditions or processes, that come about from waves transforming over a coastal profile and generating wave set up and longshore currents, will result in movement and transport of the sediments (e.g. sand) present in the profile. This is referred to as ‘’littoral transport processes’’ and is the main subject of this article. However, transport of fine sediments will also be discussed, but only very briefly.

Sediment transport, General

The sediment on the seabed is transported when it is exposed to large enough forces, or ‘’shear stresses’’, by the water movements. These movements can be caused by the current or by the wave orbital velocities or a combination of both, the latter being the most important situation. The relevant parameters for the description of the sediment transport along a shoreline or in a coastal area are therefore the following:

• The wave conditions at the site and the possible variations over the site plus the adjoining areas
• The current conditions as well as the variations of these over the area
• The water-level conditions, i.e. tide, storm surge and wave set-up
• The bathymetry (the depth variations) in the area
• The sediment characteristics over the area
• The sources and sinks of sediment, such as rivers, eroding coasts or tidal inlets

Onshore and Offshore Transport and Equilibrium Coastal Profile

Varying wave conditions result in varying onshore and offshore transports over the coastal profile. These transports are, to some extent, reversible and therefore non critical in terms of long term coastal stability. However, extreme storm surge and wave exposure result in coastal erosion.

Erosion and rebuilding sequence

When the coastal profile is exposed to non extreme waves and storm surge, the sediments near the shoreline will be transported offshore and typically be deposited in a bar resulting in an overall flattening of the slope of the shoreface. However, the inner part of the shoreface as well as the foreshore will become steeper in this process, and the shoreline will recede. During the following periods of smaller waves, swell and normal water-level conditions, the bar will travel very slowly towards the coastline again, practically rebuilding the original coastal profile.

During such a sequence of profile erosion and rebuilding, certain parts of the coastal profile may experience temporary erosion. This may not be recorded in profile surveys, because some rebuilding will already have taken place before it is possible to carry out surveys after the storm. It is important to take such temporary profile fluctuations into account when designing structures in the coastal zone. It is particularly important to have a sufficiently wide beach so that the temporary beach erosion will not cause erosion of the coast.

Coastal profile

This onshore and offshore transport is closely related to the form of the coastal profile. Several investigations have revealed that a coastal profile possesses an average, characteristic form, which is referred to as the theoretical equilibrium profile. The equilibrium profile has been defined as “a statistical average profile, which maintains its form apart from small fluctuations, including seasonal fluctuations.” The depth d [meters] in the equilibrium profile increases exponentially with the distance x from the shoreline according to the equation ():

[math]Insert formula here[/math] d = A xm [x and d in meters]

where A is the dimensionless steepness parameter and m is a dimensionless exponent. Based on fitting to natural upper shoreface profiles, Dean has suggested an average value of m = 0.67. However the value of m is subject to large variability dependent of the beach type expressed by the dimensionless fall velocity Ω = H0/ωsT, where H0 is the deep water wave height, T is the wave period and ωs is the sediment fall velocity. The value of m varies typically between m ~ 0.4 for reflective beaches (Ω < 1.5) and m ~ 0.8 for dissipative beaches (Ω > 5.5). ().

The steepness parameter A has empirically been related () to the sediment fall velocity ωs as follows:

[math]Insert formula here[/math] A = 0.067ωs0.44 [ωs in cm s-1]

Values for A as a function of the mean grain size d50 is shown in the table below.

Table 6.2 Correlation between mean grain size d50 in mm and the constant A in Dean’s equilibrium profile equation.

d50 0.10 0.15 0.20 0.25 0.30 0.50 1.00 2.00 5.00 10.00 A 0.043 0.062 0.080 0.092 0.103 0.132 0.178 0.234 0.318 0.390

It is seen that the equilibrium profile does not depend on the wave height. The reason for this is that the water depth limits the wave height inside the breaker zone. However, the wave height decides the width of the littoral zone, within which the equilibrium shoreface concept is valid. Thus, the equilibrium profile is only valid for the littoral zone, i.e. out to the Closure Depth dl:

as defined in Section 3.

The width of the littoral zone and the slope of the shoreface thus depend on the mean grain size as well as on the wave conditions.

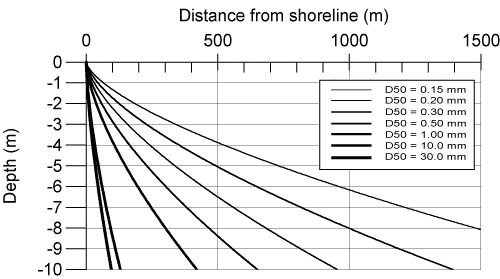
The equilibrium profile becomes increasingly steeper with increasing grain size. Typical equilibrium profiles for different grain size characteristics are presented in the following figure.

Note: For the width of the littoral zone, see Fig 6.6.

Equilibrium profiles for grain sizes 0.15, 0.2, 0.3, 0.5, 1.0, 10 and 30 mm.

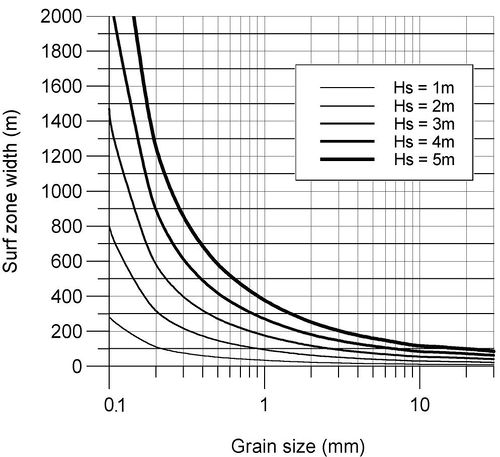
The width of the littoral zone as a function of the mean grain size and for different wave climates, represented by HS, 12 h/y, is presented in the following figure.

Empirical width of littoral zone as a function of the mean grain size for various wave climates represented by HS, 12 h/y.

These figures can be used in preliminary design considerations for artificial beaches and reclamation areas fronted by natural slopes.

It is evident from these correlations between grain size, equilibrium profile and wave conditions that it is very important in beach nourishment to use materials as coarse as or coarser than the native material. Otherwise the nourished sand will immediately be transported offshore in nature’s attempt to form the new and flatter equilibrium profile, which fits the finer sand, see also Section 12.5.

In the real world there is often a sorting of the sediments in the active coastal profile; the mean grain size decreases with increasing distance from the shoreline. If this variation is introduced into the considerations concerning the equilibrium profile, a more accurate representation of the equilibrium profile at a specific site is obtained.

The concept of equilibrium profiles is a rather crude representation of the coastal profile conditions since it neither includes nor explains the occurrence of bar formations, etc. However, the concept of the equilibrium profile is a rather practical “tool” for the analysis of coastal conditions and, as already mentioned, for preliminary design considerations.

If the geological coastal profile at a location is flatter than the calculated equilibrium profile, the wave action in the profile will tend to form the equilibrium profile, which means that material will be moved towards the shore. However, at a certain location towards the shore, there is not sufficient wave energy to move the sand any further and a barrier with a corresponding lagoon is formed.

The equilibrium concept can also explain why shore and coast erosion take place at locations where the equilibrium profile is already established, when such profiles are exposed to the combined action of storm waves and storm surge (tidal wave). The increased water level will correspond to a profile, which is too steep compared to the equilibrium profile. At a certain distance from the shoreline the water will consequently be too deep relative to the equilibrium depth. Nature will compensate by transporting sand from the beach towards the sea in an attempt to re-establish the equilibrium profile, which fits the temporary high water level. This will result in setback of the shoreline; however, if the beach is not sufficiently wide for this adjustment, the sediment will be taken from the cliff or dunes. The amount of erosion during a storm thus depends primarily upon the magnitude of the storm surge and its duration. It is evident from this description that a wide beach is a precondition for a stable coastline. Coast protection can thus be established by providing a wide beach through beach or foreshore nourishment. After the storm, the material, which was brought offshore during the storm surge conditions, will to a great extent be transported slowly back to the beach, however extreme storm surge/wave events will result in a permanent offshore loss of material.